UNIVERSITÄT ZU KÖLN

Stefanie Walch

We carry out radiative transfer simulations with the 3D Monte-Carlo radiative transfer code RADMC-3D to compute the radial momentum injected into different clouds (homogeneous and fractal with different widths and mean surface densities) by radiation pressure. The method takes into account isotropic scattering and absorption, as well as the associated shift to longer wavelengths upon re-emission of photons. In this way, a given input spectrum quickly changes from a single-peaked, black-body like shape to a double-peaked profile with pronounced UV and IR bumps. This result only depends on the dust density and on the dust species and is thus applicable for different gas metallicities if one assumes a constant scaling of gas metallicity and dust-to-gas ratio.

We modified the RADMC-3D code to record the radiation pressure acquired by every cell in the computational domain. We then carry out radiative transfer simulations for central massive stars and different central star clusters irradiating homogenous and fractal molecular clouds with different masses. We vary the mass of the cloud such that a certain gas surface density is obtained. Different dust models are also explored, where the one we show here is the typical Milky Way model from Weingartner & Draine. 

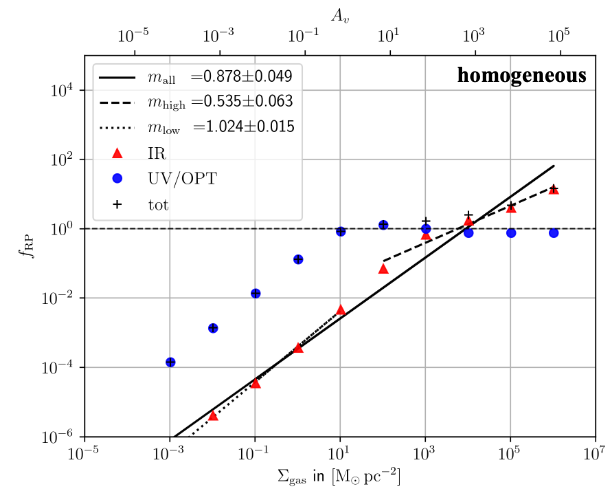
In each model, we place the same massive star in the center of a molecular cloud with varying conditions. The clouds have different substructures from homogeneous (no substructure) to very clumpy (wide fractal) and different surface densities, Σ_gas.

The plots shows the radial momentum gained due to the radiation pressure on dust, normalised to the bolometric luminosity of the central source over the speed of light. This corresponds to the so-called boost-factor, f_RP. It can be larger than unity due to multiple scattering in case the surface density becomes high enough such that the cloud becomes optically thick in the infrared. We show the results for homogeneous clouds (Fig. 1) and for clouds which have a clumpy, fractal substructure (Fig. 2). For the fractal case, we test different random realisations by changing the random seed and the fractal dimension. We split up the total radial momentum (black crosses) into the contribution from first absorption of UV and optical light photons (blue circles) and the contribution from re-processed infrared (IR) radiation (red triangles). For clouds with mean surface densities of 10³ M_☉ pc^-2 and above, the IR contribution dominates and causes the radial momentum to increase above 1 L/c due to multiple scattering in the IR. For clouds with very high surface densities, this can lead to a significant boost of the radial momentum received due to radiation pressure on dust. For clouds with conditions typical for solar neighbourhood, the IR contribution is negligible. 

We take away two important points: (1) for high surface densities, f_RP ∝ Σ_gas^0.5 ∝ τ_IR^0.5 in homogeneous media due to the redshifting of re-processed radiation. (2) Higher boost factors can be achieved in clumpy media (see Fig. 2) because they have more dense regions and also denser regions for the same amount of dust mass in the cloud. This is a different interpretation than the reduced boost factor which is generally derived for clumpy media.